Acoustic emission characteristics of Brazilian test for low-porosity sandstone under different load conditions
-
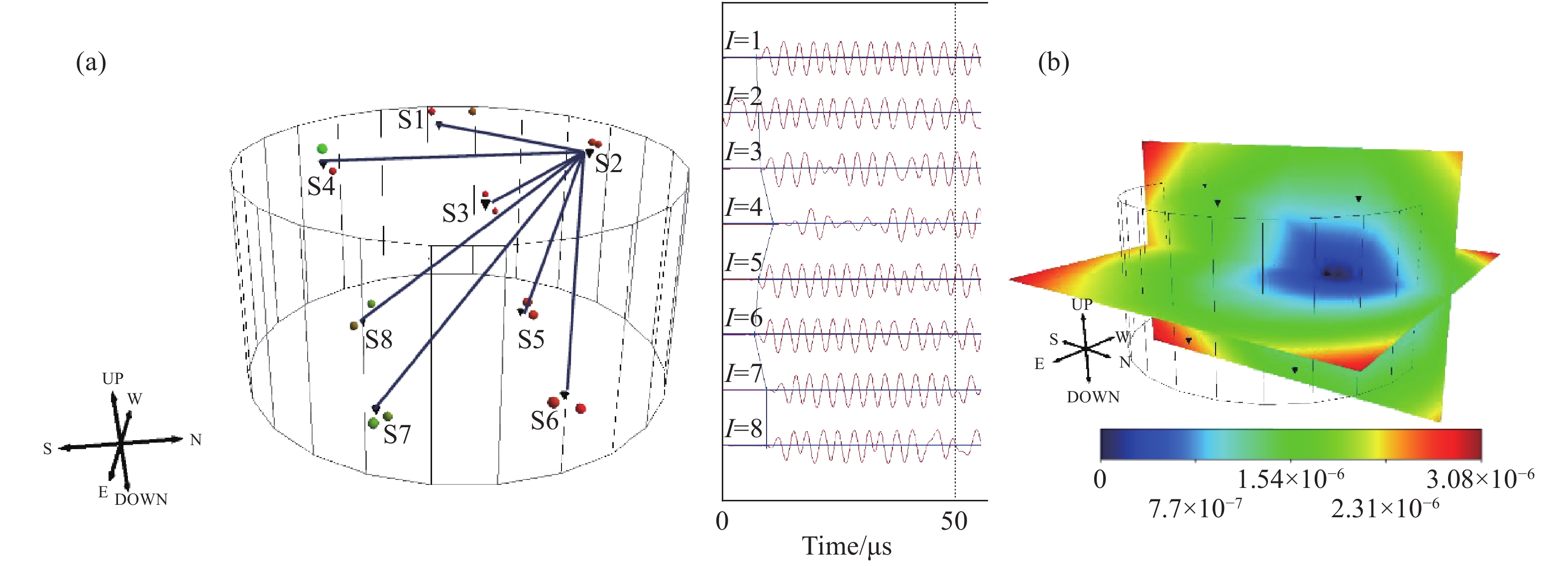
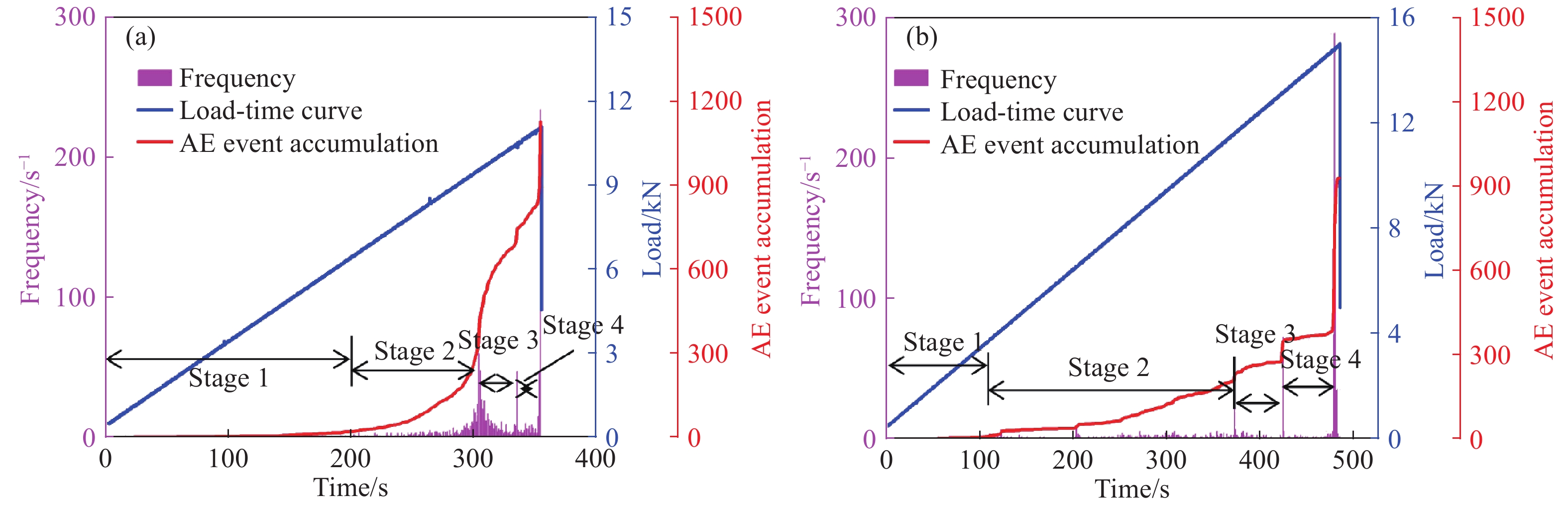
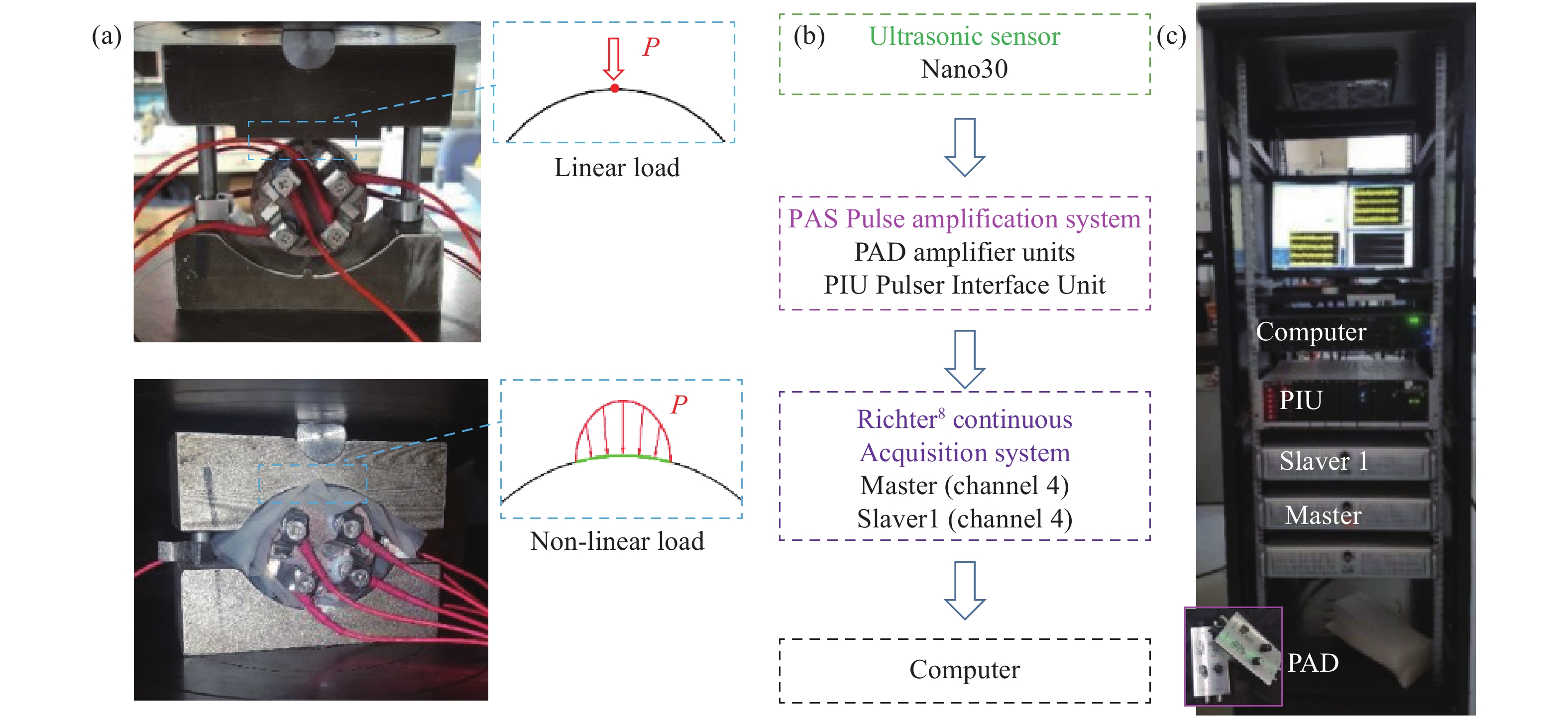
摘要: 針對巴西圓盤荷載接觸條件對巴西劈裂試驗影響的問題，采用聲發射監測系統開展線/非線荷載接觸條件下低孔隙率砂巖巴西劈裂試驗。直徑為50 mm，厚度為25 mm的標準巴西圓盤按照同一種傳感器三維布設方式布置8個Nano30傳感器。在相同的荷載速率下，聲發射監測Richter8系統對線/非線荷載兩種荷載條件下的巴西圓盤進行準靜態加載的波形信號連續記錄。通過P波自動到時及網格坍塌搜索算法進行定位，在線/非線荷載條件下分別有1131和931個聲發射事件被成功定位。圓盤的起裂位置均在圓盤非中心位置，對于非中心起裂的試驗值可能低估了巴西抗拉強度。裂紋下半球極點密度投影分析表明，非線荷載條件下破裂面的局部扭曲程度大于線荷載。試樣三維損傷演化結果表明，圓盤所受荷載面積大小，顯著影響圓盤損傷累計的時間、釋放能量的大小和裂紋擴展的穩定性。對有效聲發射定位事件進行矩張量分解獲取了兩種荷載條件下各向同性部分（ISO）、純雙力偶（DC）和補償線性矢量偶極成分（CLVD）頻率百分比，并采用微裂紋破裂類型分類方法來定量分析震源機制，結果表明巴西劈裂對荷載條件并不敏感，兩者均可以解釋為近似平行于荷載方向上的張拉裂紋的萌生、擴展及貫通。Abstract: In view of the influence of the load contact conditions on Brazilian test results, the acoustic emission (AE) monitoring system was used to conduct a Brazilian test of hard and brittle low-porosity sandstone under linear/non-linear load contact conditions. The standard Brazilian discs with a diameter of 50 mm and a thickness of 25 mm were instrumented with a three-dimensional sensor array containing eight Nano30 sensors. All the discs were equipped with identical three-dimensional sensor arrays. At the same load rate, the Brazilian discs were quasi-statically loaded under both linear/non-linear loads. The Richter 8 acquisition system continuously recorded waveform signals from eight channels from load application to brittle failure. Under the linear/non-linear load conditions, 1131 and 931 AE events were successfully located by a P-wave automatic picking and collapsing grid search algorithm. Under the linear/non-linear load condition, the crack initiation points were both away from the disc center. For non-central crack initiation, the tensile strength test may underestimate the true value. A pole density analysis of the planes under nonlinear load conditions shows that the local distortion of the fracture is greater than that under linear load. The evolution of the 3D damage to the disc shows that the load area of the disc significantly affects the cumulative time of damage, amount of energy liberation and stability of the crack propagation. The moment tensor decomposition was performed on the effective AE events, and the isotropic (ISO) component, the pure double-coupled (DC) and the compensated linear vector dipole (CLVD) component frequency percentage were obtained. The classification method was applied to quantitatively analyze the focal mechanism. The results show that the Brazilian test is not sensitive to the load contact conditions, and the focal mechanism of both cases can be interpreted as the initiation, propagation, and penetration of the tensile and shear microcracks approximately along the load direction.
-
Key words:
- Brazilian test /
- load contact condition /
- brittle failure /
- acoustic emission /
- moment tensor
-
圖 1 加載裝置及聲發射信號采集流程。（a）兩種加載方式裝置；（b）聲發射采集系統原理；（c）聲發射采集系統實物圖
Figure 1. Loading devices and acoustic emission signal acquisition setup: (a) two different loading devices; (b) schematic diagram of AE acquisition system; (c) photograph of AE acquisition system
圖 2 傳感器三維定位殘差及主動震源定位結果。（a）主動震源定位結果及傳感器2波形信號；（b）定位殘差密度切片
Figure 2. Misfit space density plane of 3D sensor array and active source locating result: (a) active source location result and the waveforms obtained through Survey 2; (b) density planes of misfit error
圖 3 荷載、聲發射事件累計數及頻率與時間的關系。（a）線荷載；（b）非線荷載
Figure 3. Variations of load, AE event accumulation and located AE rate with time: (a) linear load; (b) non-linear load
圖 4 線荷載條件下聲發射事件破裂震級及時空演化。（a）破裂震級三維視圖；（b）不同階段有效聲發射事件增量（依據信噪比繪制）
Figure 4. Located magnitude and spatial evolution of AE events for the linear load: (a) the located magnitude shown in the 3D model; (b) the effective AE increment at different stages (marker sizes are scaled by signal to noise ratio)
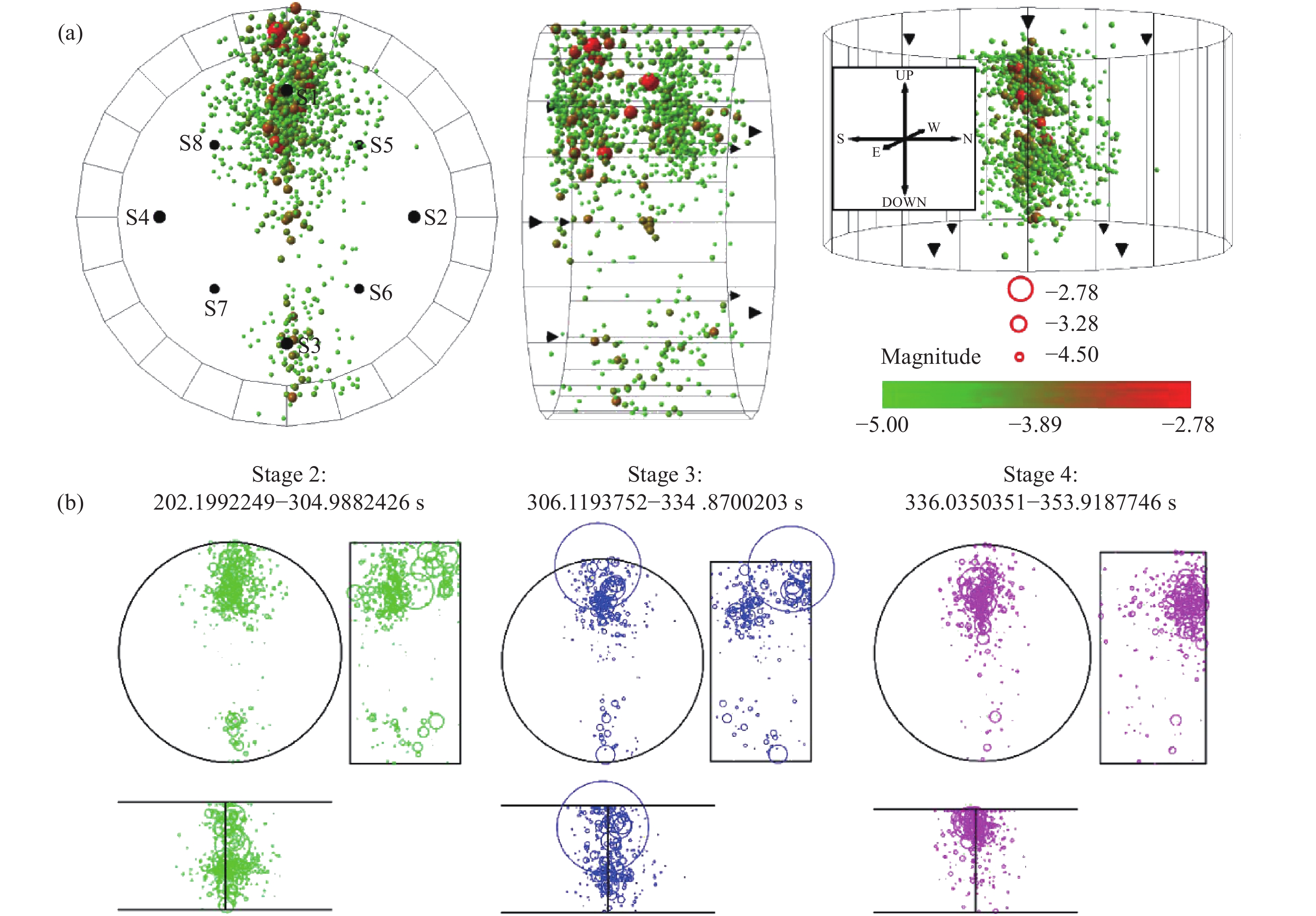
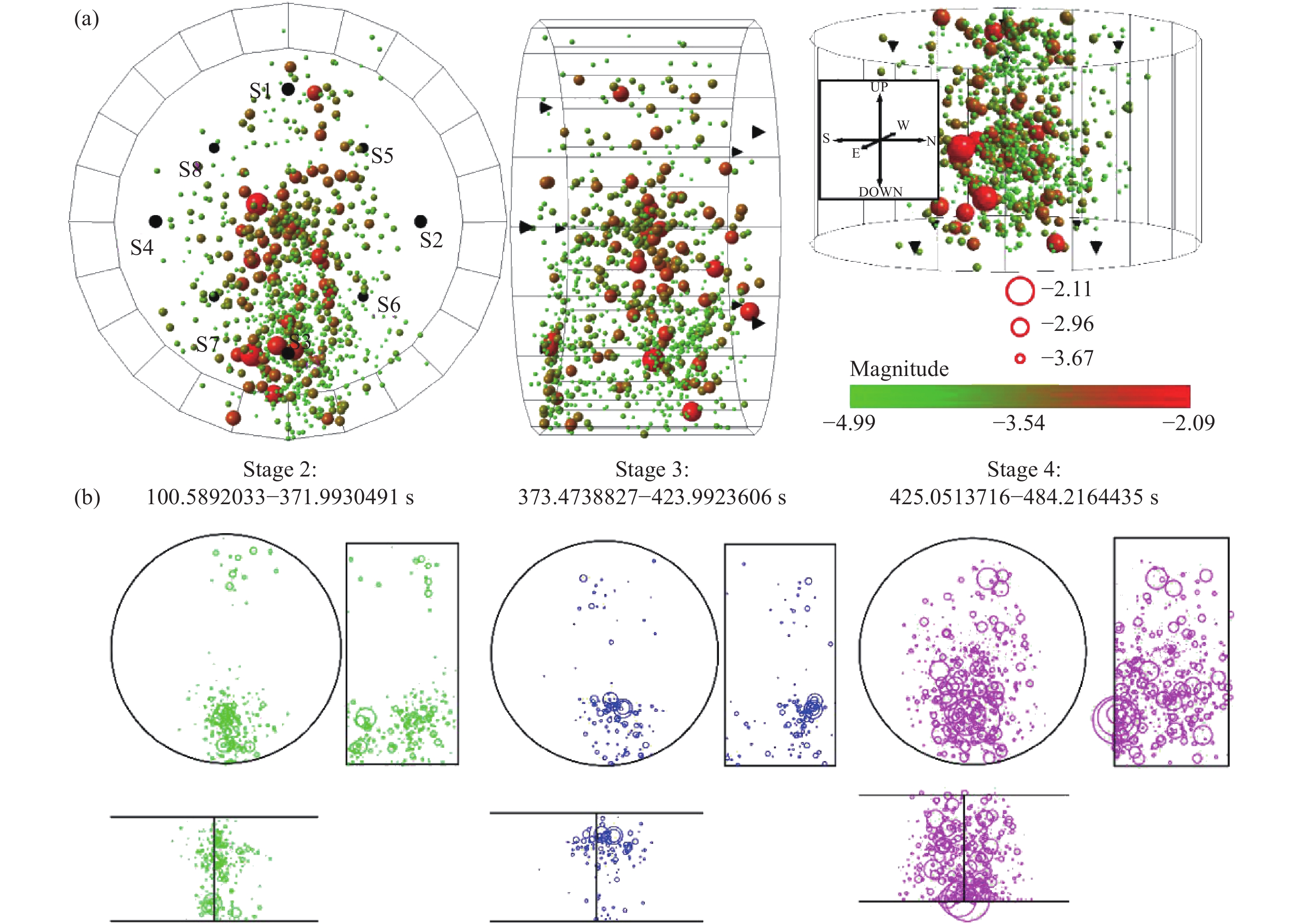
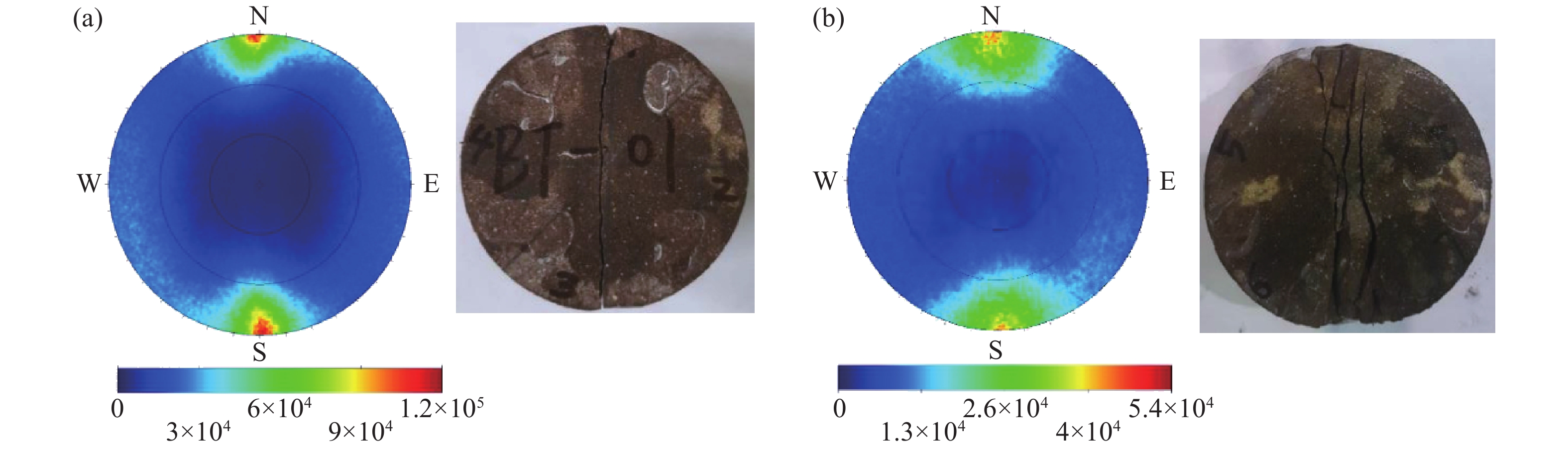
圖 5 非線荷載條件下聲發射事件破裂震級及時空演化。（a）破裂震級三維視圖；（b）不同階段有效聲發射事件增量（依據信噪比繪制）
Figure 5. Located magnitude and spatial evolution of AE events for the non-linear load: (a) the located magnitude shown in the 3D model; (b) the effective AE increment at different stages (marker sizes are scaled by signal to noise ratio)
圖 6 微裂紋極點密度及宏觀破裂面模式。（a）線荷載；（b）非線荷載
Figure 6. Stereonets of microcrack pole density and macroscopic fracture modes: (a) linear load; (b) non-linear load
圖 7 聲發射事件頻數、累計數與震級M的關系。（a）線荷載；（b）非線荷載
Figure 7. Relationship between the frequency, cumulative number and magnitude M of AE events: (a) linear load; (b) non-linear load
圖 8 矩張量成分占比。（a）線荷載；（b）非線荷載
Figure 8. Percentage of component of moment tensor components for the linear load: (a) linear load; (b) non-linear load
圖 9 震級最大的5個聲發射事件震源機制解。（a）線荷載；（b）非線荷載
Figure 9. Focal mechanism solutions of the five AE events with the largest magnitude: (a) linear load; (b) non-linear load
表 1 線/非線荷載條件下聲發射特征對比
Table 1. Comparison of acoustic emission characteristics under linear/non-linear loading
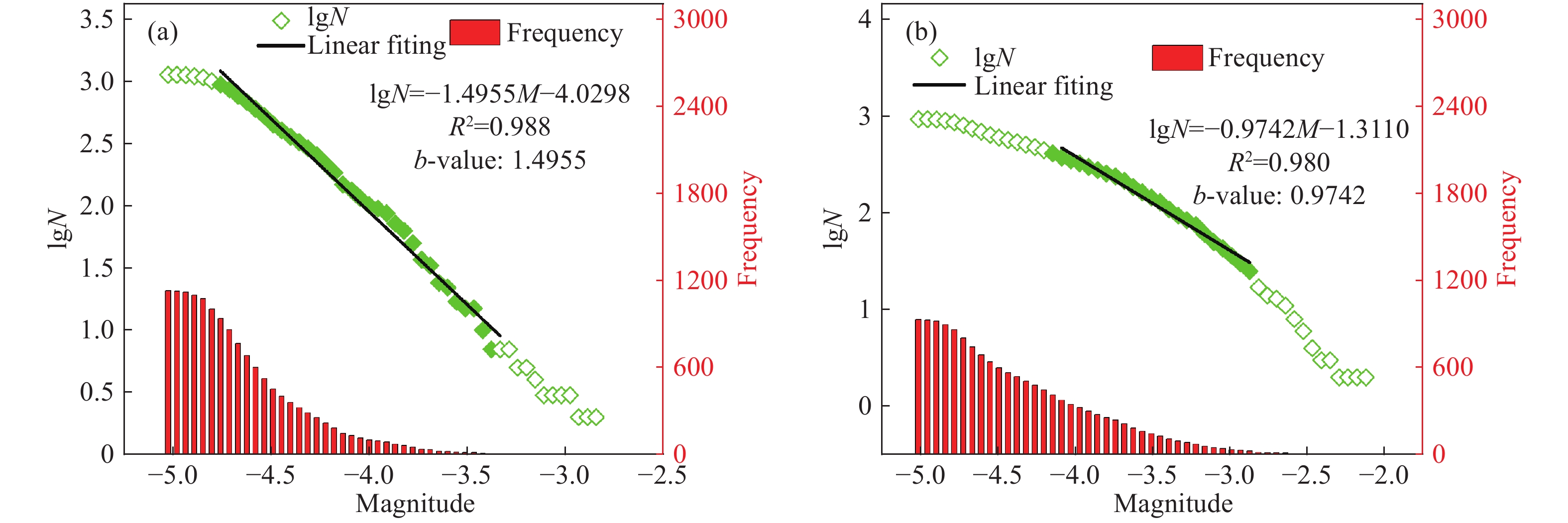
Load conditions Effective located AE events Center distance of crack initiation/mm Maximum frequency/s?1 Maximum magnitude Damage stability Strike of fracture b-value Linear load 1131 About 15 234 ?2.78 Unstable W12°N?W15°S 1.4955 Non-linear load 931 About 12 289 ?2.09 Relatively stable W18°N?W20°S 0.9742 
下載: 導出CSV
表 2 線/非線荷載條件下震源機制對比
Table 2. Comparison of focal mechanisms under linear/non-linear loads
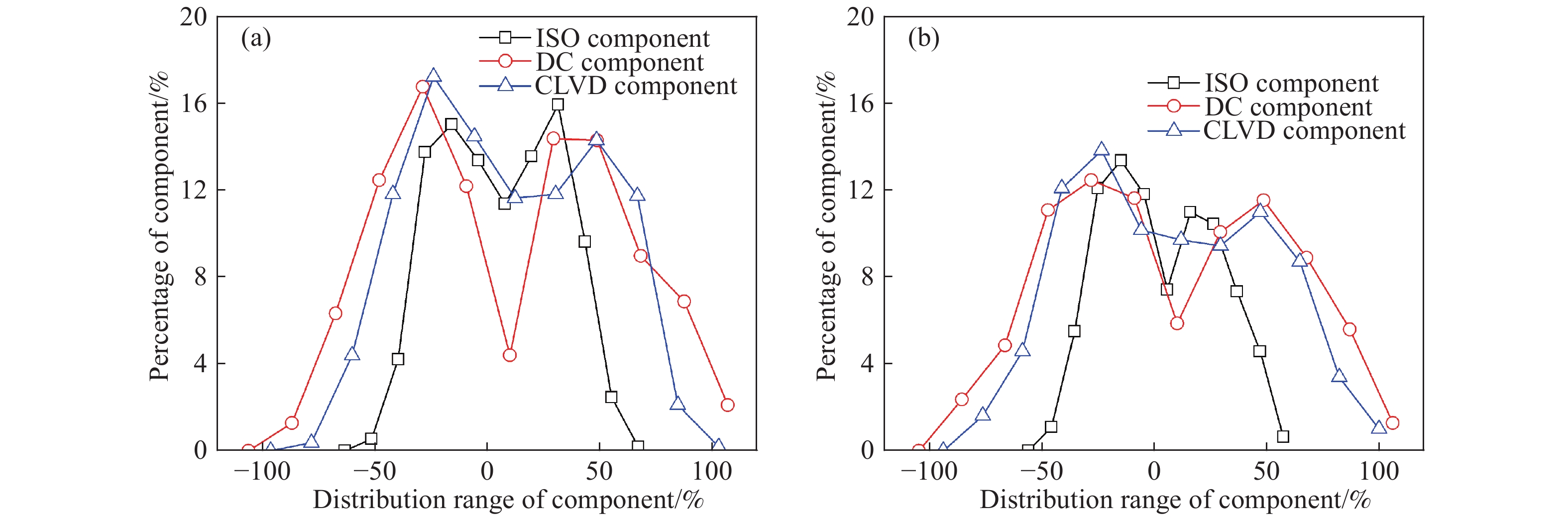
Load conditions Proportion of ISO component/% Proportion of DC component/% Proportion of CLVD component/% Proportion of tensile crack/% Proportion of shear crack/% Main source type Linear load ?40?60 ?80?100 ?60?80 47.76 24.79 Tensile and shear Non-linear load ?40?50 ?80?100 ?80?80 48.92 23.09 Tensile and shear
下載: 導出CSV
www.77susu.com<span id="fpn9h"><noframes id="fpn9h"><span id="fpn9h"></span> <span id="fpn9h"><noframes id="fpn9h"> <th id="fpn9h"></th> <strike id="fpn9h"><noframes id="fpn9h"><strike id="fpn9h"></strike> <th id="fpn9h"><noframes id="fpn9h"> <span id="fpn9h"><video id="fpn9h"></video></span> <ruby id="fpn9h"></ruby> <strike id="fpn9h"><noframes id="fpn9h"><span id="fpn9h"></span> -
參考文獻
[1] Akazawa T. New test method for evaluating internal stress due to compression of concrete (the splitting tension test) (part1). <italic>J Jpn Soc Civil Eng</italic>, 1943, 29: 777 [2] Xu X L, Wu S C, Gao Y T, et al. Effects of micro-structure and micro-parameters on brazilian tensile strength using flat-joint model. <italic>Rock Mech Rock Eng</italic>, 2016, 49(9): 1 [3] ASTM. D3967-16 Standard Test Method for Splitting Tensile Strength of Intact Rock Core Specimens. West Conshohocken: ASTM International, 2016 [4] IS RM. Suggested methods for determining tensile strength of rock materials. <italic>Int J Rock Mech Min Sci Geomech Abstr</italic>, 1978, 15(3): 99 doi: 10.1016/0148-9062(78)90003-7 [5] Ministry of Power Industry, People's Republic of China. GB/T 50266-99 Standard for Tests Method of Engineering Rock Massas. Beijing: China Planning Press, 1999中華人民共和國電力工業部. GB/T 50266-99工程巖體試驗方法標準. 北京: 中國計劃出版社, 1999 [6] Changjiang River Scientific Research Institute of Changjiang Water Resources Commission. SL264—2001 Specifications for Rock Tests in Water Conservancy and Hydroelectric Engineering. Beijing: China Water and Power Press, 2001長江水利委員會長江科學院. SL264—2001水利水電工程巖石試驗規程. 北京: 中國水利水電出版社, 2001 [7] Fairhurst C. On the validity of the ‘Brazilian’ test for brittle materials. <italic>Int J Rock Mech Min Sci Geomech Abstr</italic>, 1964, 1(4): 535 doi: 10.1016/0148-9062(64)90060-9 [8] Erarslan N, Williams D J. Experimental, numerical and analytical studies on tensile strength of rocks. <italic>Int J Rock Mech Min Sci</italic>, 2012, 49: 21 doi: 10.1016/j.ijrmms.2011.11.007 [9] Hudson J A, Brown E T, Rummel F. The controlled failure of rock discs and rings loaded in diametral compression. <italic>Int J Rock Mech Min Sci</italic>, 1972, 9(2): 241 doi: 10.1016/0148-9062(72)90025-3 [10] Lanaro F, Sato T, Stephansson O. Microcrack modelling of Brazilian tensile tests with the boundary element method. <italic>Int J Rock Mech Min Sci</italic>, 2009, 46(3): 450 doi: 10.1016/j.ijrmms.2008.11.007 [11] Markides C F, Kourkoulis S K. The stress field in a standardized Brazilian disc: the influence of the loading type acting on the actual contact length. <italic>Rock Mech Rock Eng</italic>, 2012, 45(2): 145 doi: 10.1007/s00603-011-0201-2 [12] Garcia-Fernandez C C, Gonzalez-Nicieza C, Alvarez-Fernandez M I, et al. Analytical and experimental study of failure onset during a Brazilian test. <italic>Int J Rock Mech Min Sci</italic>, 2018, 103: 254 doi: 10.1016/j.ijrmms.2018.01.045 [13] King M S, Pettitt W S, Haycox J R, et al. Acoustic emissions associated with the formation of fracture sets in sandstone under polyaxial stress conditions. <italic>Geophys Prospect</italic>, 2012, 60: 93 doi: 10.1111/j.1365-2478.2011.00959.x [14] Chow T, Hutchins D A, Falls S D, et al. Ultrasonic attenuation tomography in disks under load//IEEE Symposium on Ultrasonics. Honolulu, 1990 [15] Wang Y S, Deng J H, Li L R, et al. Micro-failure analysis of direct and flat loading Brazilian tensile tests. <italic>Rock Mech Rock Eng</italic>, 2019, 52(11): 4175 doi: 10.1007/s00603-019-01877-7 [16] Wu S C, Guo P, Zhang S H, et al. Study on thermal damage of granite based on Brazilian splitting test. Chin J Rock Mech Eng, 2018, 37(Suppl 2): 3805吳順川, 郭沛, 張詩淮, 等. 基于巴西劈裂試驗的花崗巖熱損傷研究. 巖石力學與工程學報, 2018, 37(增刊 2): 3805 [17] Liu X L, Liu Z, Li X B, et al. Acoustic emission b-values of limestone under uniaxial compression and Brazilian splitting loads. Rock Soil Mech, 2019, 40(Suppl 1): 267劉希靈, 劉周, 李夕兵, 等. 單軸壓縮與劈裂荷載下灰巖聲發射b值特性研究. 巖土力學, 2019, 40(增刊 1): 267 [18] Falls S D. Ultrasonic Imaging and Acoustic Emission Studies of Microcrack Development in Lac du Bonnet Granite[Dissertation]. Canada: Queen's University at Kingston, 1993 [19] Zhang S H, Wu S C, Zhang G, et al. Three-dimensional evolution of damage in sandstone Brazilian discs by the concurrent use of active and passive ultrasonic techniques. <italic>Acta Geotech</italic>, 2020, 15(2): 393 doi: 10.1007/s11440-018-0737-3 [20] Ren H L, Ning J G, Song S Z, et al. Investigation on crack growth in concrete by moment tensor analysis of acoustic emission. <italic>Chin J Theoret Appl Mech</italic>, 2019, 51(6): 1830 doi: 10.6052/0459-1879-19-170任會蘭, 寧建國, 宋水舟, 等. 基于聲發射矩張量分析混凝土破壞的裂紋運動. 力學學報, 2019, 51(6):1830 doi: 10.6052/0459-1879-19-170 [21] Zhang S W, Xian X F, Zhou J P, et al. Acoustic emission characteristics and the energy distribution of the shale in Brazilian splitting testing. J China Coal Soc, 2017, 42(Suppl 2): 346張樹文, 鮮學福, 周軍平, 等. 基于巴西劈裂試驗的頁巖聲發射與能量分布特征研究. 煤炭學報, 2017, 42(增刊 2): 346 [22] Zhang S H, Wu S C, Chu C Q, et al. Acoustic emission associated with self-sustaining failure in low-porosity sandstone under uniaxial compression. <italic>Rock Mech Rock Eng</italic>, 2019, 52(7): 2067 doi: 10.1007/s00603-018-1686-8 [23] Zhang S H. Study on Strength and Deformability of Hard Brittle Sandstone[Dissertation]. Beijing: University of Science and Technology Beijing, 2019張詩淮. 硬脆性砂巖強度與變形特性研究[學位論文]. 北京: 北京科技大學, 2019 [24] Fehler M, House L, Kaieda H. Determining planes along which earthquakes occur: method and application to earthquakes accompanying hydraulic fracturing. <italic>J Geophys Res Solid Earth</italic>, 1987, 92(B9): 9407 doi: 10.1029/JB092iB09p09407 [25] Gutenberg G, Richter C F. Seismicity of the earth and associated phenomena. <italic>J Geophys Res</italic>, 1950, 55: 97 doi: 10.1029/JZ055i001p00097 [26] Knopoff L, Randall M J. The compensated linear-vector dipole: a possible mechanism for deep earthquakes. <italic>J Geophys Res</italic>, 1970, 75(26): 4957 doi: 10.1029/JB075i026p04957 [27] Finck F, Kurz J H, Grosse C U, et al. Advances in moment tensor inversion for civil engineering//International Symposium on Non-Destructive Testing in Civil Engineering, 2003 [28] Ohtsu M. Simplified moment tensor analysis and unified decomposition of acoustic emission source: application to in situ hydrofracturing test. <italic>J Geophys Res Solid Earth</italic>, 1991, 96(B4): 6211 doi: 10.1029/90JB02689 [29] Zhang Q, Zhang X P. The crack nature analysis of primary and secondary cracks: a numerical study based on moment tensors. <italic>Eng Fract Mech</italic>, 2019, 210: 70 doi: 10.1016/j.engfracmech.2018.05.006 [30] Vavry?uk V, Kühn D. Moment tensor inversion of waveforms: a two-step time-frequency approach. <italic>Geophys J Int</italic>, 2012, 190(3): 1761 doi: 10.1111/j.1365-246X.2012.05592.x [31] Dai F, Jiang P, Xu N W, et al. Focal mechanism determination for microseismic events and its application to the left bank slope of the Baihetan hydropower station in China. <italic>Environ Earth Sci</italic>, 2018, 77(7): 268 doi: 10.1007/s12665-018-7443-1 [32] Yu Y. Questioning the validity of the Brazilian test for determining tensile strength of rocks. <italic>Chin J Rock Mech Eng</italic>, 2005, 24(7): 1150 doi: 10.3321/j.issn:1000-6915.2005.07.011喻勇. 質疑巖石巴西圓盤拉伸強度試驗. 巖石力學與工程學報, 2005, 24(7):1150 doi: 10.3321/j.issn:1000-6915.2005.07.011 [33] Li D Y, Wong L N Y. The Brazilian disc test for rock mechanics applications: review and new insights. <italic>Rock Mech Rock Eng</italic>, 2013, 46(2): 269 doi: 10.1007/s00603-012-0257-7 [34] Komurlu E, Kesimal A. Evaluation of indirect tensile strength of rocks using different types of jaws. <italic>Rock Mech Rock Eng</italic>, 2015, 48(4): 1723 doi: 10.1007/s00603-014-0644-3 [35] Erarslan N, Liang Z Z, Williams D J. Experimental and numerical studies on determination of indirect tensile strength of rocks. <italic>Rock Mech Rock Eng</italic>, 2012, 45(5): 739 [36] Guo X, Wang X B, Bai X Y, et al. Numerical simulation of effects of loading types and tensile strengths on Brazilian disk test by use of a continuum-discontinuum method. <italic>Rock Soil Mech</italic>, 2017, 38(1): 214郭翔, 王學濱, 白雪元, 等. 加載方式及抗拉強度對巴西圓盤試驗影響的連續?非連續方法數值模擬. 巖土力學, 2017, 38(1):214 -
點擊查看大圖
計量
- 文章訪問數: 2194
- HTML全文瀏覽量: 1134
- PDF下載量: 61
- 被引次數: 0
